Assignment 4 was handed out in class. You can see a copy here with the additional solutions here.
I had a question about some of the solutions.
In Question 4(g), why does ![]() as an exponent necessitate using
as an exponent necessitate using ![]() as a denominator.
as a denominator.
It is because ![]()
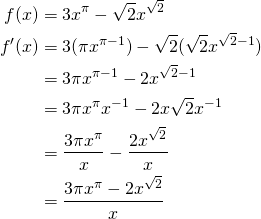
Tags: assignment, Calculus, MAE 113
-
Thanks for this, makes sense now. Would this be for any variable? Ex. y-1 as exponent?
2 comments
Comments feed for this article
Trackback link: https://kohar.ca/mae-113-calculus-for-liberal-arts-winter-2018/assignment-4/trackback/